Special Right Triangle: 30º-60º-90º MathBitsNotebook.com Topical Outline | Geometry Outline | MathBits' Teacher Resources Terms of Use Contact Person:Donna Roberts |
Although all right triangles have special features – trigonometric functions and the Pythagorean theorem. The most frequently studied right triangles, the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 triangles. The 30, 60, 90 Special Right Triangle. The Math section of the SAT is full of special right triangles. The 5-12-13 triangle is a common triple. If you can recognize the triple pattern then you can easily calculate a missing side. Here is a list of the common triples associated with a 5 -12-13 right triangle.
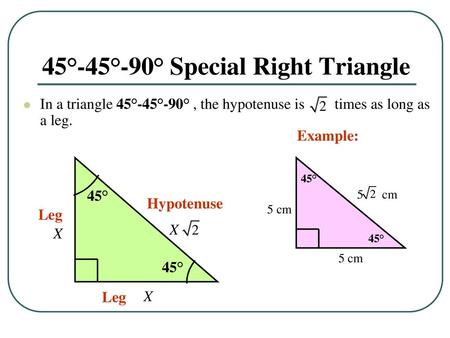
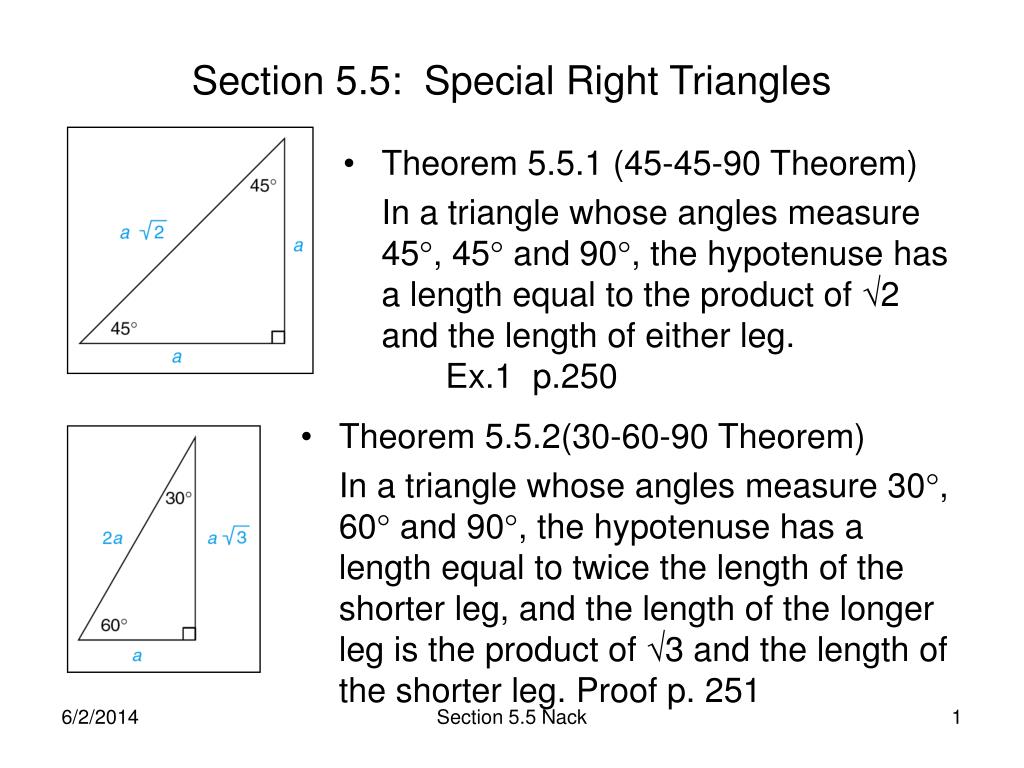
There are two 'special' right triangles that will continually appear throughout your study of mathematics: the 30º-60º-90º triangle and the 45º-45º-90º triangle. The special nature of these triangles is their ability to yield exact answers instead of decimal approximations when dealing with trigonometric functions. This page will deal with the 30º-60º-90º triangle.
All 30º-60º-90º triangles are similar! They satisfy Angle -Angle (AA) for proving trianlges similar. |
Congruent 30º-60º-90º triangles are formed when an altitude is drawn in an equilateral triangle. Remember that the altitude in an equilateral triangle will bisect the angle and is the perpendicular bisector of the side. If the side of the equilateral triangle is set to a length of 2 units, the Pythagorean Theorem will find the length of the altitude to be units. | |
Note: the side of the equilateral triangle need not be a length of 2 for the patterns to emerge. The choice of a side length of 2 simply makes the calculations easier. Yes, is smaller than 2, making the hypotenuse of 2 the longest side. Remember 2 can be written as .
| |
Once the sides of the 30º-60º-90º triangle are established, a series of relationships (patterns) can be identified between the sides of the triangle. ALL 30º-60º-90º triangles will possess these same patterns. These relationships will be referred to as 'short cut formulas' that can quickly answer questions regarding side lengths of 30º-60º-90º triangles, without having to apply any other strategies such as the Pythagorean Theorem or trigonometric functions. |
Since 30º-60º-90º triangles are similar, their corresponding sides are proportional. As such, we can establish a pattern as to how their sides are related. The following pattern formulas will let you quickly find the sides of a 30º-60º-90º triangle even when you are given only ONE side of the triangle. Remember, these formulas work ONLY in a 30º-60º-90º triangle!
|
|
Examples: |
This example shows the easiest application of the patterns (where the hypotenuse is given).
Always look at what is 'given' and what you need to find.
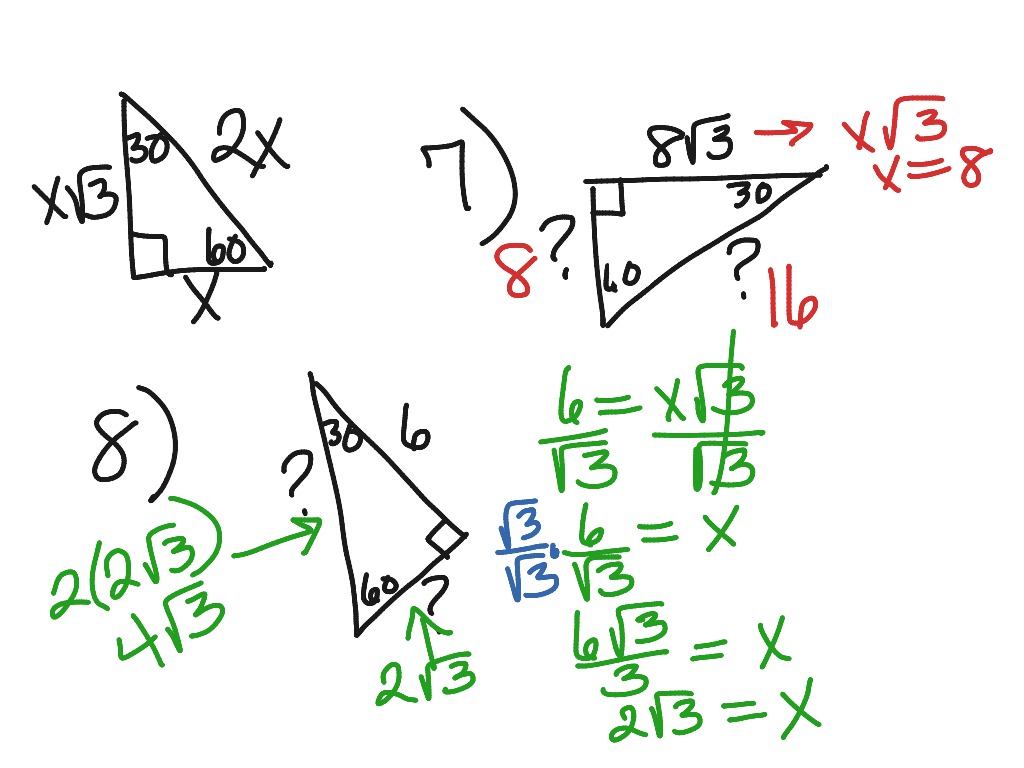
Find x and y. | x is the short leg (across from the 30º angle)x = ½ • 16 x = 8Answer | y is the long leg (across from the 60º angle)y = ½ • 16 • y = 8 Answer |
This example requires more thinking and more algebraic work (where the short leg is given).
Always start with what is 'given' and work from that point.
Find x and y. | 7 is the short leg (x is the hypotenuse) 7 = ½ • x x = 14 Answer | y is the long leg (use hypotenuse you found)y = ½ • 14 • y = 7 Answer |
This example requires the most algebraic and radical work (where long leg is a given integer).
Since the 'given' is the long leg, start with the long leg.
Find x and y. To remove a radical value from a denominator, multiply (top and bottom) by that radical value. Radical Review | y is the short leg (use hypotenuse you found)
|
Notice that when you are working with a 30º-60º-90º triangle you are working with. Look at all of the THREEs at work! 30, 60, 90, When you work with 30º-60º-90º and 45º-45º-90º triangles, you will need to keep straight which radical goes with which triangle. |
I forgot the formula patterns! Now what? Remember that there is always more than one way to attack a problem! If you forget these formula patterns, you can use trigonometry functions and/or the Pythagorean Theorem to find the missing sides. Be warned, however, that the Pythagorean Theorem by itself, will not help you find both missing sides, since the Pythagorean Theorem needs two sides to work. |
NOTE: There-posting of materials(in part or whole) from this site to the Internet is copyright violation and is not considered 'fair use' for educators. Please read the 'Terms of Use'. |
Topical Outline | Geometry Outline | MathBitsNotebook.com | MathBits' Teacher Resources
Terms of Use Contact Person: Donna Roberts
As we know, a right triangle is a triangle whose one angle always measures 90°. The longest side opposite of a right triangle is called the hypotenuse, while the horizontal leg is called the base and the vertical leg is called the height or the altitude.
What is a Special Right Triangle
Special Right Triangles Quizlet
Special right triangles are right triangles whose angles or sides are in a particular ratio. They have some regular features that make calculations on it much easier.
In geometry, the Pythagorean Theorem is commonly used to find the relationship between the sides of a right triangle, given by the equation: a2 + b2 = c2, where a, b denotes the height and base of the triangle and c is the hypotenuse. But, if it is a special right triangle, we can use simpler formulas.
Some common special right triangles are shown below.
Types of Special Right Triangle
The two most common special right triangles are:
45-45-90 Triangle
A 45-45-90 triangle is a special right triangle whose three angles measure 45°, 45° and 90°. The ratio of its side lengths (base: height: hypotenuse) is 1: 1: √2.
30-60-90 Triangle
A 30-60-90triangle is a special right triangle whose three angles measure 30°, 60° and 90°. The ratio of its side lengths (base: height: hypotenuse) is1: √3: 2.
Apart from the above two types, there are some other special right triangles.
Others: Pythagorean Triples
Some right triangles have sides that are of integer lengths and are collectively called the Pythagorean triples. Such triangles can be easily remembered and any multiple of the sides produces the same relationship. Pythagorean triples can be of three types:
- Common Pythagorean triples: Sides with integer lengths. Examples – 3-4-5, 5-12-13, 8-15-17, 7-24-25, and 9-40- 41.
- Almost-isosceles Pythagorean Triples: Sides with integer lengths but almost isosceles. Examples – 20-21-29, 119- 120-169, 696-697-985, and 4,059-4,060-5,741.
- Sides that are in Geometric Progression: Also known as the Kepler triangle, if the sides are in geometric progression a, ar, ar2, its common ratio r is given by r = √φ where φ is the golden ratio.
Formulas
Although there is no common formula for special right triangles, each of them has specific formulas for finding the missing sides, area, and perimeter based on the ratio of their side lengths. Find their formulas with solved examples in our separate articles.
How to Solve Special Right Triangles
Special Right Triangles Practice
Solving special right triangles is about finding the missing lengths of the sides. Instead of using the Pythagorean Theorem, we can simply use the special right triangle ratios to find the missing length. Let us understand the concept better by doing some practice problems.
The hypotenuse of a 45-45-90 triangle is 12√2 mm. Calculate the length of its base and height.
As we know,
Ratio of their Side Lengths = x: x: x√2, here x√2 = hypotenuse = 12√2 mm
Thus,
x√2 = 12√2 mm
Squaring both sides we get,
⇒ (x√2)2 = (12√2)2 mm
⇒ 2x2 = 144 x 2 = 288
⇒ x = √144 = 12 mm
Hence, the base and height of the given right triangle measure 16.97 mm each.
The longer side of a 30-60-90 right triangle is given by 5√3 cm. What is the measure of its shorter side and hypotenuse?

Special Right Triangles 45 45 90
As we know,
Ratio of their Side Lengths = x: x√3: 2x, here x = shorter side, x√3 = longer side = 5√3 cm, 2x = hypotenuse
Thus,
x√3 = 5√3
Squaring both sides we get,
(x√3)2 = (5√3)2
⇒ 3x2 = 25 x 3
⇒ x2 = 25
⇒ x = 5 cm
Special Right Triangles Video
The length of the hypotenuse and the other side of a right triangle are 30 cm and 24 cm, respectively. Find the length of the missing side.
As we know,
Here we have to find whether the sides are in the ratio of 3x: 4x: 5x
Thus,
?: 24: 30 = ?: 4(6): 5(6)
Thus, the sides are in the ratio of 3x: 4x: 5x and it is a 3-4-5 triangle.
For calculating the third side,
For, n = 6
Hence, the length of the other side,
3x = 3 x 6 = 18
Therefore the length of the missing side is 18 cm.
Special Right Triangles
If the two sides of a right triangle are 3 ft and 4 ft, find the length of hypotenuse.
Special Right Triangles 2
As we know,
Here we have to find whether the sides are in the ratio of 3x: 4x: 5x
3: 4: ? = 3(1): 4(1): ?
Thus, the sides are in the ratio of 3x: 4x: 5x and it is a 3-4-5 triangle
For calculating the hypotenuse,
For, n = 1
Hence, the length of the hypotenuse,
5x = 5 x 1 = 5
Therefore the length of the hypotenuse is 5 ft